Least Squares Regression Line

2 9 5 7 8 8 9 2. We start with a collection of points with coordinates given by x i y i.

Y A Bx Stats Chapter 5 Least Squares Regression Linear Relationships Regression High School Math

Any straight line will pass among these points and will either go above or below each of these.

. The reader can download the line-spacing measurement data as a text file Model Types and Weighted Least Squares. Suppose we wanted to estimate a score for someone who had spent exactly 23 hours on an essay. The statsmodelapi allows us to fit an Ordinary Least Squares model.

We can calculate the distances from these points to. Ordinary Least Squares OLS linear regression is a statistical technique used for the analysis and modelling of linear relationships between a response variable and one or more predictor variables. We can change our layout a bit so its more manageable.

In statistics ordinary least squares OLS is a type of linear least squares method for estimating the unknown parameters in a linear regression model. The least squares regression line is one such line through our data points. The least squares method is a form of mathematical regression analysis that finds the line of best fit for a dataset providing a visual demonstration of the relationship.

At least three values are needed so we can take any kind of information our of the graph Adding some style. Regression is a statistical measure used in finance investing and other disciplines that attempts to determine the strength of the relationship between one dependent variable usually denoted by. In a room full of people youll notice that no two lines of best fit.

The Linear Regression model attempts to find the relationship between variables by finding the best fit line. Putting these elements together we get that. Enter your data as x y pairs and find the equation of a line that best fits the data.

The partial least squares regression is the extension of the PCR method which does not suffer from the mentioned deficiency. The least squares regression line is the line that best fits the data. It is the statistical way of measuring the relationship between one or more independent variables vs one dependent variable.

Use Method of Least Squares to Fit Regression Line. Given any collection of pairs of numbers except when all the x-values are the same and the corresponding scatter diagram there always exists exactly one straight line that fits the data better than any other in the sense of. This calibration must be done.

The graphical plot of linear regression line is as follows. Im sure most of us have experience in drawing lines of best fit where we line up a ruler think this seems about right and draw some lines from the X to the Y axis. If the relationship between two variables appears to be linear then a straight line can be fit to the data in order to model the relationship.

First lets create the following dataset in Excel. Least Squares Method. Least squares regression line example.

In statistics linear regression is a linear approach to modelling the relationship between a dependent variable and one or more independent variables. The second one position one is for our regression line. Least Squares Regression Line of Best Fit.

OLS chooses the parameters of a linear function of a set of explanatory variables by the principle of least squares. Minimizing the sum of the squares of the differences between the observed dependent variable values of the variable. The following step-by-step example shows how to use this function in practice.

So what does the least squares really mean. We minimize the sum of squared differences between actual observations of the dependent variable vs. Least-angle regression is an estimation procedure for linear regression models that was developed to handle high-dimensional covariate vectors potentially with more covariates than observations.

Int gsl_fit_mul const double x const size_t xstride const double y const size_t ystride size_t n double c1 double cov11 double sumsq This function computes the best-fit linear regression coefficient c1 of. In the case of one independent variable it is called simple linear regression. Our free online linear regression calculator gives step by step calculations of any regression analysis.

部分的最小二乗回帰 Partial Least Squares Regression PLS についてpdfとパワーポイントの資料を作成しましたデータセットが与えられたときにPLSで何ができるかどのようにPLSを計算するかが説明されています. PLS acronym of Partial Least Squares is a widespread regression technique used to analyse near-infrared spectroscopy data. The formula for the line of the best fit with least squares estimation is then.

Unlike linear and nonlinear least squares regression weighted least squares regression is not associated with a particular type of function used to describe the relationship between the process variables. These parameters are chosen and estimated by the method of least squares eg. Nothing major it just serves as.

Imagine you have some points and want to have a line that best fits them like this. If you know a bit about NIR spectroscopy you sure know very well that NIR is a secondary method and NIR data needs to be calibrated against primary reference data of the parameter one seeks to measure. Linear Least-Squares Fitting.

The name of the least squares line explains what it does. By Theorem 1 of One Sample Hypothesis Testing for Correlation under certain conditions the test statistic t has the property. But for better accuracy lets see how to calculate the line using Least Squares Regression.

Linear Regression is one of the most important algorithms in machine learning. We have to grab our instance of the chart and call update so we see the new values being taken into account. The functions described in this section can be used to perform least-squares fits to a straight line model without a constant term.

Find the least squares regression line for the data set as follows. The Least Squares Regression Line. The slope β 1 of the least squares regression line estimates the size and direction of the mean change in the dependent variable y when the independent variable x is increased by one unit.

Also work for the estimated value of y for the value of X to be 2. Linear regression where the sum of vertical distances d1 d2 d3 d4 between observed and predicted line and its equation. The least squares regression line is the line that minimizes the sum of the squares d1 d2 d3 d4 of the vertical deviation from each data point to the line see figure below as an example of 4 points.

For more than one independent variable the process is called mulitple linear regression. Least Squares Regression is a way of finding a straight line that best fits the data called the Line of Best Fit. Try to have the line as close as possible to all points and a similar number of points above and below the line.

The least-squares regression line is the line that makes the sum of the squares of the vertical distances of the data points from the line as small as possible these vertical distances from each data point to the least-squares regression line are called the residual values. To use the method of least squares to fit a regression line in Excel we can use the LINEST function. But by Property 1 of Method of Least Squares.

We now show how to test the value of the slope of the regression line. Y a x b. We can place the line by eye.

Its slope and y-intercept are computed from the data using formulas. Our aim is to. The TheilSen estimator is a simple robust estimation.

This applet lets you explore how the correlation and least-squares regression line changes as points are. The magic lies in the way of working out the parameters a and b. This is a linear model that estimates the intercept and regression coefficient.

And by Definition 3 of Regression Analysis and Property 4 of Regression Analysis. As you can see the least square regression line equation is no different that the standard expression for linear dependency.

Linear Regression Using Least Squares Method Line Of Best Fit Equation Youtube Linear Regression Numerical Methods Line Of Best Fit

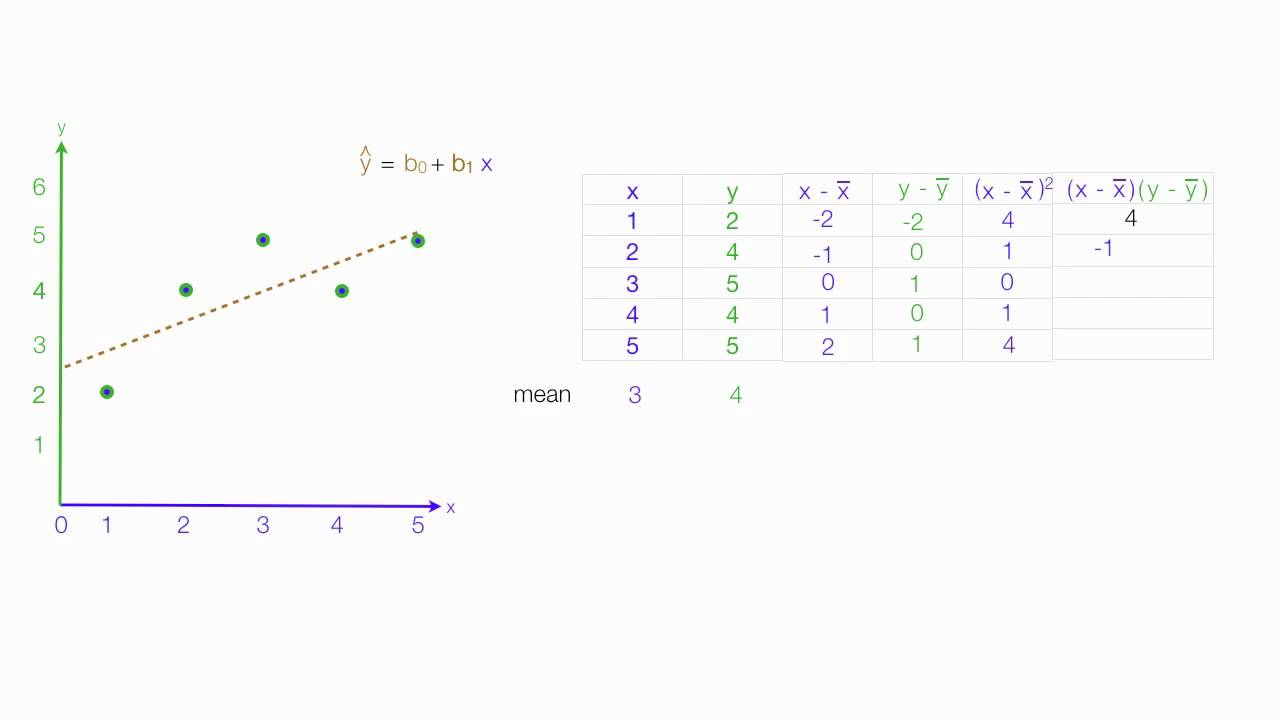
How To Calculate Linear Regression Using Least Square Method

Least Squares Regression Analysis Can Help Projects Regression Analysis Statistics Math Machine Learning Deep Learning

How To Calculate Least Squares Regression Line Regression Calculator Square